NEWSLETTER December 1997
University Approval for the Clarkson Road Project
 A small group of us began work on the project late in 1992. We had three main tasks before us: fund-raising, designing the buildings, and gaining the University's formal support and financial commitment to the project, fundraising and designing the buildings. The third of these was completed on 20 June 1997 when the Regent House, the body composed of University academic staff, formally gave authority for a planning application to be submitted to the Cambridge City Council. We hope that the application will be approved before the end of this year.
A small group of us began work on the project late in 1992. We had three main tasks before us: fund-raising, designing the buildings, and gaining the University's formal support and financial commitment to the project, fundraising and designing the buildings. The third of these was completed on 20 June 1997 when the Regent House, the body composed of University academic staff, formally gave authority for a planning application to be submitted to the Cambridge City Council. We hope that the application will be approved before the end of this year.
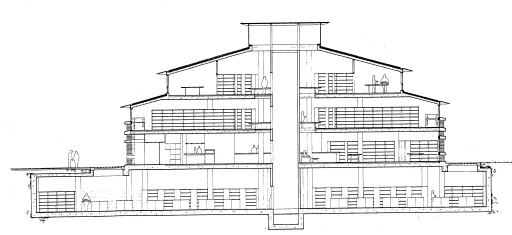
Meanwhile, we have been hard at work with the design team. Edward Cullinan Architects have produced imaginative and exciting designs, and we hope to begin work next March and complete the first phase of the building before the end of 1999.
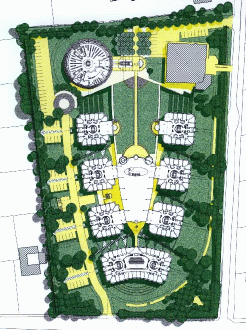
The evolution of the plans has lead to the removal of the double pavilion next to the circular library building, which has been moved into the space made (see plan below).
We have almost enough money for half of the buildings intended for the two mathematics departments. Our external supporters have generously pledged more than 8 million pounds, and nearly 10 million pounds has been committed from within Cambridge. A further 2 million pounds is needed for the most cost-effective construction of the first phase, due to begin in March 1998. It is now a particularly crucial time in our fund-raising: pledges of even small sums can make a big difference.
Further information
For further information about the Clarkson Road Project, contact
Professor Peter Landshoff
fax: +44 (0)1223 337918
e-mail: pvl@damtp.cam.ac.uk
A new world-class science library
Our original intention was simply to move the two University mathematics departments onto the Clarkson Road site, to join the Isaac Newton Institute for Mathematical Sciences, a national institute which since 1992 has been the sole occupant of the site. However, early in 1993 we began negotiations with the University Librarian also to set up a new library on the site. These negotiations began with the modest aim of alleviating the problems of the Scientific Periodicals Library, which is under severe pressure for space on its central site. However, following University-wide discussions it was finally agreed that a major new library will be established on the site. It will formally be part of the main University Library, which is one of the six copyright deposit libraries in the British Isles. The new library will house books as well as periodicals, and will cover mathematics, the physical sciences, and technology.
Peter Fox, the University Librarian, says: "The proposed Physical Sciences and Technology Library at Clarkson Road will be a major world-class new library. It is designed to meet the needs of library users on the day it opens and also will be flexible enough to allow the University to respond to changes in modes of access to information. Almost all those concerned with thinking about and planning the information provision of the future are convinced that the notion of the fully electronic library and thus the elimination of the library as a place, is a myth. Even in the sciences, the library will remain a hybrid - retaining traditional books and journals alongside electronic resources - for a very long time.
There will probably be a gradual change in space use as the need for good quality, high-technology study space increases and as the output of paper journals declines. An increasing amount of information will be made available electronically, either directly to the scholar from services such as e-print archives or via intermediaries. Even in the physical sciences and technology, significant use is made of older articles, which are not available electronically. The major journals will probably be scanned and digitised over a period of years, but many will not, and the library's traditional role of providing access to such journals will remain. A study of scientific and technical publishing carried out in 1997 by the Royal Society and others indicated a concern that electronic publishing made the holding of national collections of hard-copy journals for current and archival use even more essential. As a legal deposit library, Cambridge's role in this will be crucial and the holdings at Clarkson Road will form part of the national collection in purpose-built accommodation of imaginative design."
Archival Materials
by Anthony Edwards,
Reader in Biometry in the Department of Community Medicine,
and Chairman of the Library Syndicate.
 When Henry Lucas died in 1663 he not only provided for the establishment of the Lucasian Professorship of Mathematics but bequeathed his library of 4,000 volumes to the University, thus initiating a special relationship between the University Library and the Professorship which still flourishes today. Sir Isaac Newton was the second holder of the Professorship, and amongst the manuscript treasures of the Library are the Newton papers, including his discovery of the general binomial theorem, whilst the present holder, Professor Stephen Hawking, has donated to the Library the typescript of A Brief History of Time. Lucas's books include Galileo's Dialogo of 1632, a harbinger of a collection of mathematical books now without comparison in the English-speaking world. As a library of legal deposit, the University Library contains copies of every important mathematical text published in the British Isles, and gifts, bequests, and purchases of earlier printed material over the centuries have ensured that scholars have available, usually in several editions, all the important early books in mathematics and probability. Notable collections given to the Library are those of John Venn (1889), inventor of the Venn diagram, John Couch Adams (1892), co-discoverer of Neptune, and F.P. White (1969), which contains some 1,200 books from the 16th to the 18th century. Amongst its early manuscripts the Library counts a fine twelfth-century De Arithmetica of Boethius, and a very early Arithmetical Triangle from a Chinese manuscript of 1407 in which the circles containing the Chinese numerals have been printed rather than drawn. (There are two copies of Pascal's Trait‚ du triangle arithm‚tique, 1665, in the Library.)
When Henry Lucas died in 1663 he not only provided for the establishment of the Lucasian Professorship of Mathematics but bequeathed his library of 4,000 volumes to the University, thus initiating a special relationship between the University Library and the Professorship which still flourishes today. Sir Isaac Newton was the second holder of the Professorship, and amongst the manuscript treasures of the Library are the Newton papers, including his discovery of the general binomial theorem, whilst the present holder, Professor Stephen Hawking, has donated to the Library the typescript of A Brief History of Time. Lucas's books include Galileo's Dialogo of 1632, a harbinger of a collection of mathematical books now without comparison in the English-speaking world. As a library of legal deposit, the University Library contains copies of every important mathematical text published in the British Isles, and gifts, bequests, and purchases of earlier printed material over the centuries have ensured that scholars have available, usually in several editions, all the important early books in mathematics and probability. Notable collections given to the Library are those of John Venn (1889), inventor of the Venn diagram, John Couch Adams (1892), co-discoverer of Neptune, and F.P. White (1969), which contains some 1,200 books from the 16th to the 18th century. Amongst its early manuscripts the Library counts a fine twelfth-century De Arithmetica of Boethius, and a very early Arithmetical Triangle from a Chinese manuscript of 1407 in which the circles containing the Chinese numerals have been printed rather than drawn. (There are two copies of Pascal's Trait‚ du triangle arithm‚tique, 1665, in the Library.)
In addition to the Newton papers the Library has significant collections of the papers of physicists Clerk Maxwell, Rutherford, J.J. Thompson, G.G. Stokes and Lord Kelvin, as well as the extensive archive of the Royal Greenwich Observatory, whilst sets of the complete works of all major mathematicians are available on the open shelves of the Library.
Codes and Cryptography
by Richard Pinch, lecturer in Pure Mathematics at Queens' College.
His research interests are in computational number theory and its application to cryptography.
Making messages easy to read
 On the first day after the Pathfinder mission landed the Rover on Mars, the NASA World-Wide Web sites recorded over a hundred million 'hits' on images which had come over tens of millions of miles from a transmitter sending out a signal about as powerful as a domestic light bulb. These digital signals have to be protected against corruption from the much stronger interference generated by the Sun by error-correcting codes. One obvious way of doing this would be to repeat each message over and over again - but this would slow down unbearably the rate of transmission.
On the first day after the Pathfinder mission landed the Rover on Mars, the NASA World-Wide Web sites recorded over a hundred million 'hits' on images which had come over tens of millions of miles from a transmitter sending out a signal about as powerful as a domestic light bulb. These digital signals have to be protected against corruption from the much stronger interference generated by the Sun by error-correcting codes. One obvious way of doing this would be to repeat each message over and over again - but this would slow down unbearably the rate of transmission.
Codes which combine enough redundancy to compensate for errors with sufficient speed of transmission were first developed at Bell Laboratories in the 1940s. The underlying mathematics was developed independently in Russia and again here in Cambridge. Theoretical limits on the best possible codes were soon developed but this work was existential: it gave no clue as to how to construct such codes in practice.
In 1995 an explicit and practical recipe for constructing these optimal codes emerged from the theory of modular curves. These geometric objects had been the subject of intense study by number theorists, and had played a crucial role in the proof of Fermat's Last Theorem by Andrew Wiles. Their appearance in this practical context was if anything even more surprising than in the work of Wiles.
Making messages hard to read
Codes in the form used by NASA are not to be confused with ciphers: ways of concealing rather than revealing information. For a long time the theory and practice of cryptography had been the exclusive preoccupation of governments, but the spread of rapid electronic communication in business and finance has led to increasing academic interest.
Conventional secret-key cipher systems, such as the famous Enigma, have been in use for many years. In their modern form, the underlying element is often a shift-register, which generates a continuous stream of data unintelligible to anyone who does not possess the initial secret key. Curiously, the theory of shift registers is formally identical to that of the optimal error-correcting codes already mentioned.
In the 1970's the concept of public-key cryptography emerged. The initial problem it resolved was that of exchanging secret information (such as the key to a conventional cipher system) between parties who could only communicate in public, for example, across an insecure network such as the Internet. The security of such a system depends on the difficulty of solving certain kinds of mathematical problem: an important example being the difficulty of resolving a large number (perhaps of hundreds of digits) into its component factors. If you know the factors, multiplying them together is easy: if you don't, finding them is hard - possession of the factors is the secret which allows the authorized recipients to decipher the message.
Difficulty
Onlookers might imagine that number theory consisted entirely of hard sums. In fact, computational problems such as factorization had early fallen out of fashion, and even after the development of modern computers, strictly computational questions were the province of a eccentric minority (such as the present author). The connexion with public-key cryptography caused an upsurge of interest, both in methods for attacking old problems and in finding new computational questions as the basis for new cryptosystems.
Back to the Dark Ages
One of my own recent interests has been in going back to some of the early methods for factorization of large numbers, including those which predate the electronic computer (when a variety of wonderful mechanical and electric devices were in use). Many practitioners have been under the impression that the old methods somehow ceased to work after the introduction of computers. It has therefore been particularly gratifying to find this work taken up by the committee drafting ANSI standards for public-key cryptography.
Closing the circle
The mathematics of elliptic curves is currently regarded as one of the most promising areas for the development of new cryptographic systems. Their particularly rich mathematical structure translates into new computational questions, but also poses the possibility of new attacks. One of the most powerful new methods for factorizing large numbers involves their use.
The theory of elliptic curves, having been developed over the years for its intrinsic interest and beauty by many workers, including a strong group here in Cambridge, was yet another of the fundamental components in the proof of Fermat's Last Theorem.
Elliptic curves stand as a splendid illustration of the unexpected connections, not only between the various branches of pure mathematics, but between pure mathematics and other disciplines.
Gift from the Garfield Weston Foundation
News has recently been received of a 2.5 million pound donation to the new Centre for Mathematical Sciences from the Garfield Weston Foundation. The gift is thanks to the support of Garfield Weston, Chairman of Associated British Foods plc and Fortnum & Mason. The Garfield Weston Foundation was established in 1958 by Garfield Weston's father with the aim of supporting a wide range of activities in the field of religion, education, the environment, the arts, and health. Garfield Weston is one of the most generous benefactor's to charitable causes in Britain and the University has previously benefited from an unearmarked donation of 1 million pounds made via the Foundation, back in 1989. The gift comes at a critical time for the Centre as it will help to enable the first phase of the building to proceed on schedule.
Friends of Mathematics at Cambridge
Following a very successful inaugural event at the Isaac Newton Institute for Mathematical Sciences, hosted by David Bell, Chairman of the Financial Times, the second meeting was held on 12 November, with talks at the Newton Institute attended by supporters and benefactors of the Centre for Mathematical Sciences. Afterwards a dinner was held at the Master's Lodge, St John's College, at which Sir Martin Rees was the speaker. A third event is planned for Tuesday 17 March 1998. If you would like to know more about the Friends, please contact Tim Simmons or Alan Watchman at the University Development Office, tel: 01223 332288, fax: 01223 460817, or email: ts205@cam.ac.uk or raw31@cam.ac.uk.
The Wolfson Foundation:
Grant towards Teaching Complex
 Following a visit to Cambridge by Lord and Lady Wolfson, an application for a grant towards the cost of the Centre for Mathematical Sciences was submitted to the Wolfson Foundation. The Foundation generously offered a grant of 1 million pounds towards the Central Teaching Complex and this has been accepted by the University. A full report will appear in the next Newsletter.
Following a visit to Cambridge by Lord and Lady Wolfson, an application for a grant towards the cost of the Centre for Mathematical Sciences was submitted to the Wolfson Foundation. The Foundation generously offered a grant of 1 million pounds towards the Central Teaching Complex and this has been accepted by the University. A full report will appear in the next Newsletter.
Institute of Actuaries
The President and Fellows of the Institute of Actuaries very kindly hosted a meeting and reception on behalf of the Faculty of Mathematics and the Centre for Mathematical Sciences on 22 October. Members of the Faculty and Institute of Actuaries, many of whom read Mathematics at Cambridge, heard talks by Professor David Crighton and Professor Geoffrey Grimmett about current developments in Pure and Applied Mathematics, and by Professor Peter Goddard about the Clarkson Road Project. Grateful thanks are due to the current President of the Institute of Actuaries, Duncan Ferguson (Trinity 1961), and the host of the event , Senior Vice-President Professor David Wilkie (Trinity 1952). At the event, Sir Brian Corby (St John's 1949) launched an appeal to name a lecture theatre in the new Centre after gifts from members of the actuarial profession and this is already halfway towards its target of 100,000 pounds.
LATE NEWS
Approval of Planning Application
On 6 January the Planning Committee of Cambridge City Council gave the go-ahead for the Centre for Mathematical Sciences. "This is excellent news," said Peter Goddard, Chairman of the Clarkson Road Working Group. "Now we should definitely be able to break ground in April this year."

